Topic : Arithmetic & Geometric Progression PYQ
FORMULAE OF ARITHMETIC & GEOMETRIC PROGRESSION
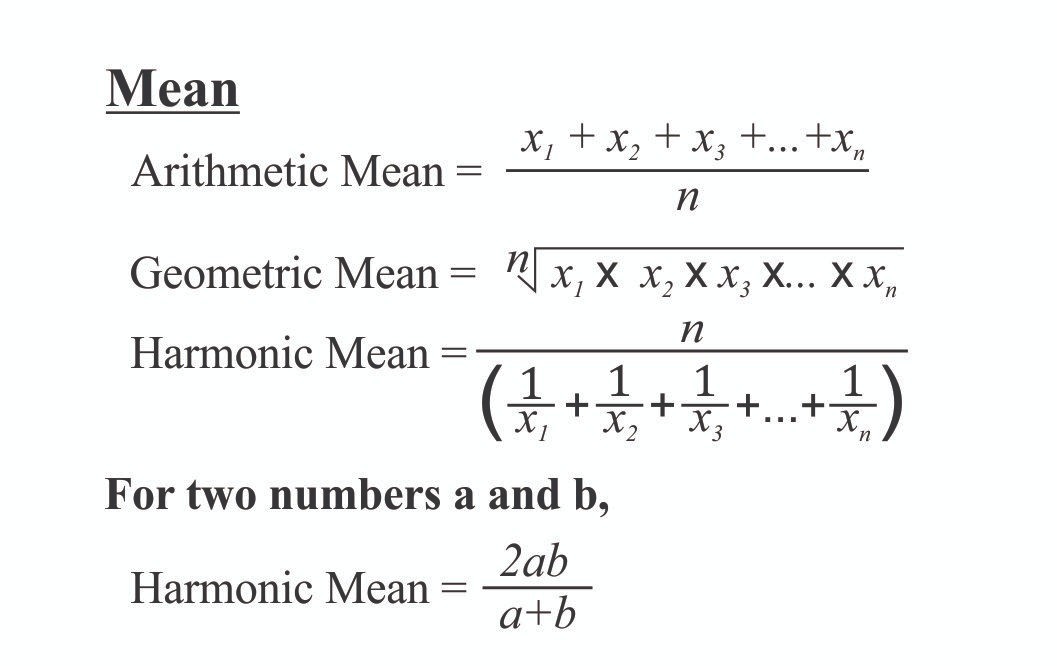
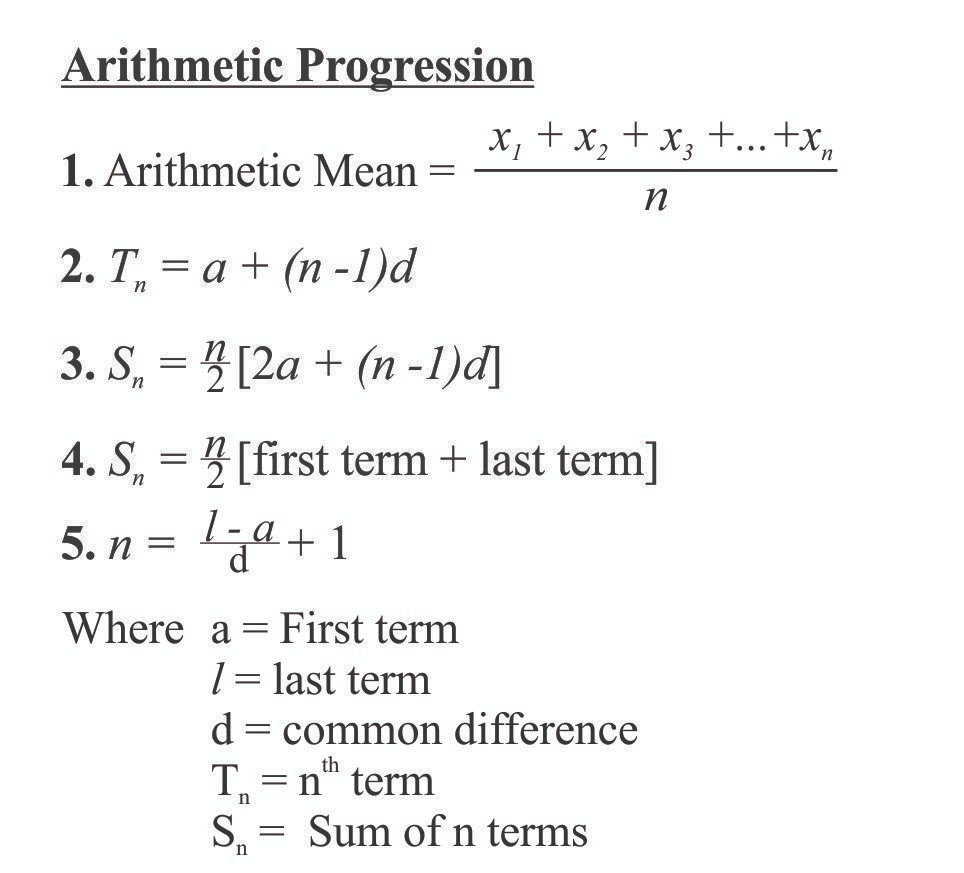
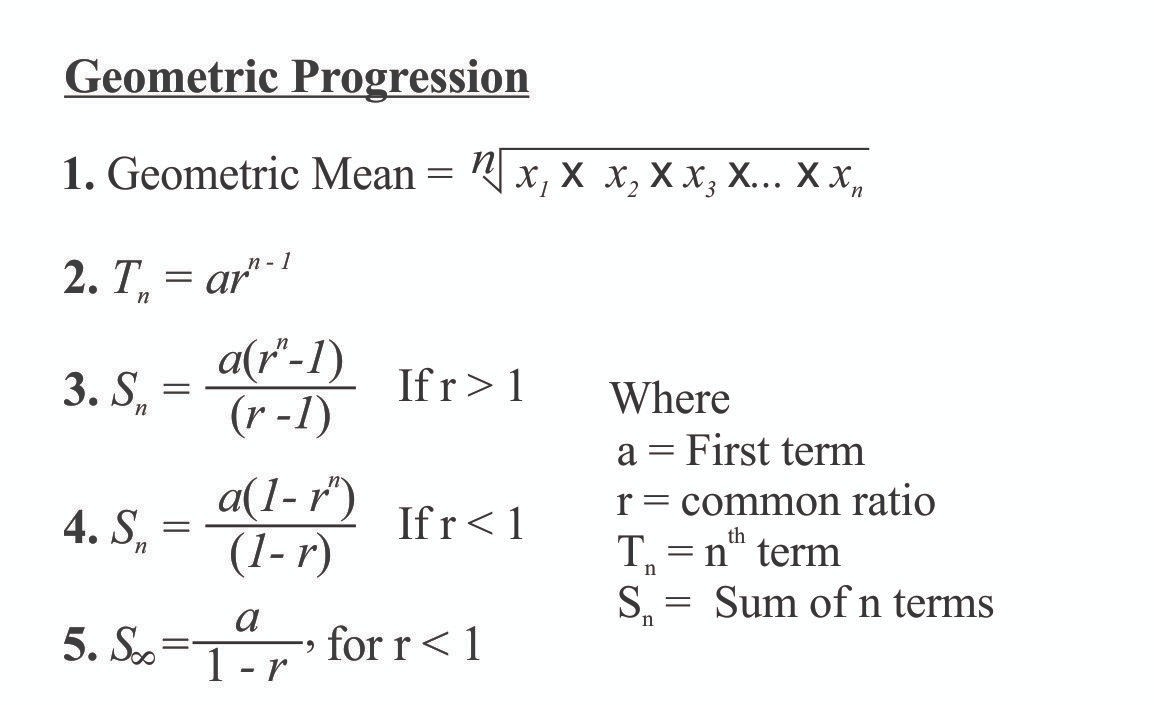
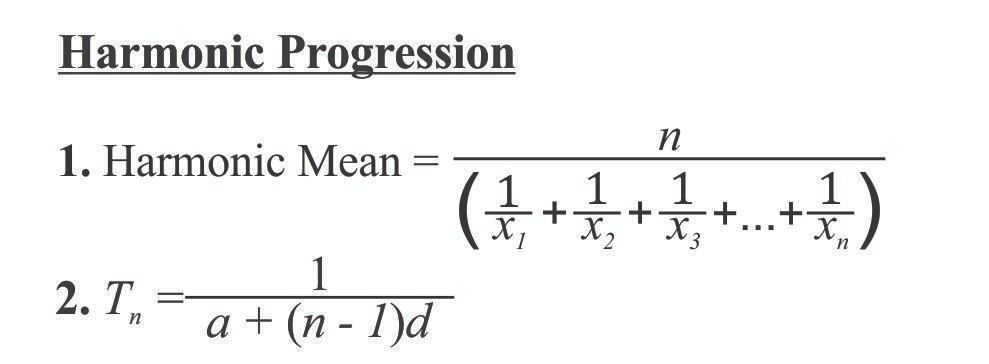
Q1. A group of 630 children is seated in rows for a group photo session. Each row contains three less children then the row in front of it. Which one of the following number of rows is not possible? [CSAT 2014]
(a) 3
(b) 4
(c) 5
(d) 6
Solution:
Given that,
A group of 630 children is seated in rows for a group photo session.
Total children seated = 630
Each row contains three less children then the row in front of it.
Let A be the number of children in 1st row
Case I: If there are three rows
A, A - 3 and A - 6
A + A - 3 + A - 6 = 630 = 3A - 9 = 630 or A = 213
Thus it is possible
Case II: 4 rows
A + A - 3 + A - 6 + A - 9 = 630 or A = 162 which is possible
Case III: 5 rows
A + A - 3 + A - 6 + A - 9 + A - 12 = 630 or A = 132 which is possible
Case IV: 6 rows
A + A - 3 + A - 6 + A - 9 + A - 12 + A - 15 = 630 or A = 112.5 (NOT POSSIBLE)
Hence option (d) is correct
Q2. There are thirteen 2-digit consecutive odd numbers. If 39 is the mean of the first five such numbers, then what is the mean of all the thirteen numbers? [CSAT 2017]
(a) 47
(b) 49
(c) 51
(d) 45
Solution:
Given that,
There are thirteen 2-digit consecutive odd numbers.
Let the number be 2x + 1, 2x + 3........2x + 25
If 39 is the mean of the first five such numbers
(2x + 1 + 2x + 3 + 2x + 5 + 2x + 7 + 2x + 9)/5 = 39
10x + 25 = 195
10x = 170
x = 17
Thus the 13 numbers are 35, 37, 39, 41......59
Sum of terms,
Sn = n/2 [first term + last term]
Sn = 13/2 [35+59]
= 611
(35 + 37 + 39....+ 59)/13 = 611/13 = 47
Hence option (a) is correct
Q3. From January 1, 2021, the price of petrol (in Rupees per litre) on mth day of the year is 80 + 0.1m where m = 1, 2, 3 ..., 100 and thereafter remains constant. On the other hand, the price of diesel (in Rupees per litre) on nth day of 2021 is 69 + 0.15n for any n. On which date in the year 2021 are the prices of these two fuels equal? [CSAT 2021]
(a) 21st May
(b) 20th May
(c) 19th May
(d) 18th May
Solution:
Given that,
The price of petrol (in Rupees per litre) on mth day of the year is 80+0.1m where m = 1 2, 3 ..., 100 and thereafter remains constant.
On 100th day the price = 80 + 0.1 x 100 = Rs.90
thereafter remains constant
On the other hand, the price of diesel (in Rupees per litre) on nth day of 2021 is 69+ 0.15n for any n.
For equal prices there are two cases
Case (i): before 100th day
80+0.1m = 69+ 0.15m (where m = n prices become equal)
m = 220 days
Thus it is not possible as m <100 days
Case (ii): m ≥ 100
As the price will remain constant
69+ 0.15m = 90
m = 140 days
Thus the date will be 20th May
Hence option (b) is correct
Q4. For five children with ages a < b < c < d < e; any two successive ages differ by 2 years.
Question: What is the age of the youngest child?
Statement-1: The age of the eldest is 3 times the youngest.
Statement-2: The average age of the children is 8 years.
Which one of the following is correct in respect of the above Question and the Statements? [CSAT 2023]
(a) The Question can be answered by using one of the Statements alone, but cannot be answered using the other Statement alone.
(b) The Question can be answered by using either Statement alone.
(c) The Question can be answered by using both the Statements together, but cannot be answered using either Statement alone.
(d) The Question cannot be answered even by using both the Statements together.
Solution:
Given that,
For five children with ages a < b < c < d < e any two successive ages differ by 2 years
Let the ages be x
X, X + 2, X + 4, X + 6 and X + 8
5X + 20.....(i)
From statement I: The age of the eldest is 3 times the youngest.
X + 8 = 3 x (X)
X = 4
Thus the ages are 4, 6, 8, 10 and 12
Hence statement I alone is sufficient to answer the question
Statement-2: The average age of the children is 8 years.
(X + X + 2 + X + 4 + X + 6 + X + 8) = 40
5X = 20 or X = 4
Thus the ages are 4, 6, 8, 10 and 12
Hence statement II alone is sufficient to answer the question
Option (b) is correct
Q5. A boy plays with a ball and he drops it from a height of 1.5 m. Every time the ball hits the ground, it bounces back to attain a height 4/5th of the previous height. The ball does not bounce further if the previous height is less than 50 cm. What is the number of times the ball hits the ground before the ball stops bouncing? [CSAT 2021]
(a) 4
(b) 5
(c) 6
(d) 7
Solution:
Given that,
Height from which ball is dropped = 1.5 m or 150 cm
Bounces 4/5th of the height every time
Does not bounce if the height is < 50cm
|
No. of drops |
Height before |
Bouncing Height |
|
1 |
150 |
(4/5) x 150 = 120 cm |
|
2 |
120 |
(4/5) x 120 = 96 cm |
|
3 |
96 |
(4/5) x 96 = 76.8 cm |
|
4 |
76.8 |
(4/5) x 76.8 = 61.44 cm |
|
5 |
61.44 |
(4/5) x 61.44 = 49.152 cm |
|
6 |
49.152 cm |
No bounce |
No. of bounces = 5
Hence option (b) is correct
Q6. What is the sum of the first 28 terms in the following sequence?
1, 1, 2, 1, 3, 2, 1, 4, 3, 2, 1, 5, 4, 3, 2,... [CSAT 2024]
(a) 83
(b) 84
(c) 85
(d) 86
Solution:
Given that,
1, 1, 2, 1, 3, 2, 1, 4, 3, 2, 1, 5, 4, 3, 2.......
Now,
Understanding the sequence
Leaving first term aside
1, 1, 2, 1, 3, 2, 1, 4, 3, 2, 1, 5, 4, 3, 2.......
Reverse order
|
Sequence |
Total sum |
|
1 |
1 |
|
2, 1 |
3 |
|
3, 2, 1 |
6 |
|
4, 3, 2, 1 |
10 |
|
5, 4, 3, 2, 1 |
15 |
|
6, 5, 4, 3, 2, 1 |
21 |
|
Total terms = 21 |
Total sum = 56 |
Now for 28 terms
Sequence would be 7, 6, 5, 4, 3, 2
not taking 1 as it will be 29th term
Sum = 27 now adding the 1st term 1 then 27+1 = 28
Total 28 term sum = 56 + 28 = 84
Hence option (b) is correct
ANSWER KEY
1. D
2. A
3. B
4. B
5. B
6. B
Related Links:
- Analytical Reasoning PYQ's
- Average PYQ
- Blood Relation PYQ's
- Boats & Streams PYQ's
- Clock PYQs for UPSC (2011-2024)
- Coding Decoding PYQs for UPSC (2011-2024)
- Continuous Series PYQ
- Cube and Dice PYQ
- Direction Sense PYQ's
- General Mental Ability PYQs for UPSC (2011-2024)
- Geometry & Mensuration PYQs for UPSC (2011-2024)
- Insert the Missing Character PYQs for UPSC (2011-2024)
- Mixture & Alligation PYQ's
- Number Ranking & Sequence PYQs for UPSC (2011-2024)
- Number System PYQs for UPSC (2011-2024) | Solved Questions & PDF
- Venn Diagrams PYQs for UPSC (2011-2024)
- PDF's & Booklets
- UPSC Previous Year Question Papers
- Calendar PYQs for UPSC (2011-2024)
- Data Interpretation PYQs for UPSC (2011-2024)
- Mixture & Alligation PYQs for UPSC (2011-2024)

